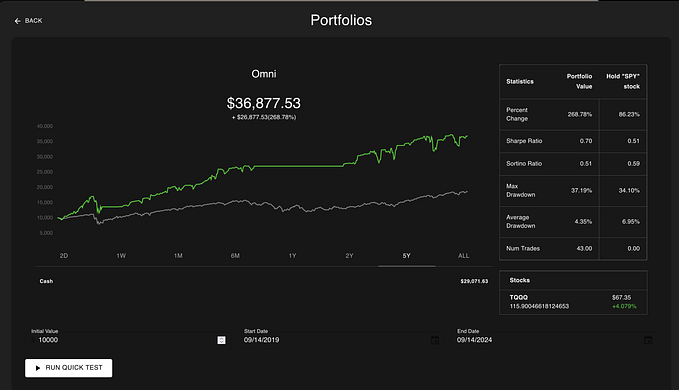
Those of you who’ve seen my slide deck, perhaps with an eye to performing it yourself, per my suggestion-invitation, may recall that slide wherein I go:
4D: Coxeter (polytopes, extended Euclideanism, perpendiculars)
4D: Einstein (3D space + imaginary time)
4D: RBF (a container as a concept primitively “4-eyed”)
I’m paraphrasing, working from memory. I’ll screenshot the slide in question below. [1] RBF = R. Buckminster Fuller, grand nephew of Margaret Fuller, the New England transcendentalist (Emerson, Whitman…).

Many a phony intellect, e.g. an AI chatter box, might not care that the “tesseract as time machine” meme comes from science fiction. Shades of Flatland (also science fiction).
Chatbots interweave namespaces, indulge in name collisions, as a means of achieving verisimilitude. How could a bot competently simulate bureaucratic talk, without an ability to deliberately obfuscate?
The tesseract belongs squarely with the Euclideans and is most often considered timeless, whereas the “time machine” meme is perennially a part of Atomic Age physics (which the hippies…